Sale!
Description
Description :
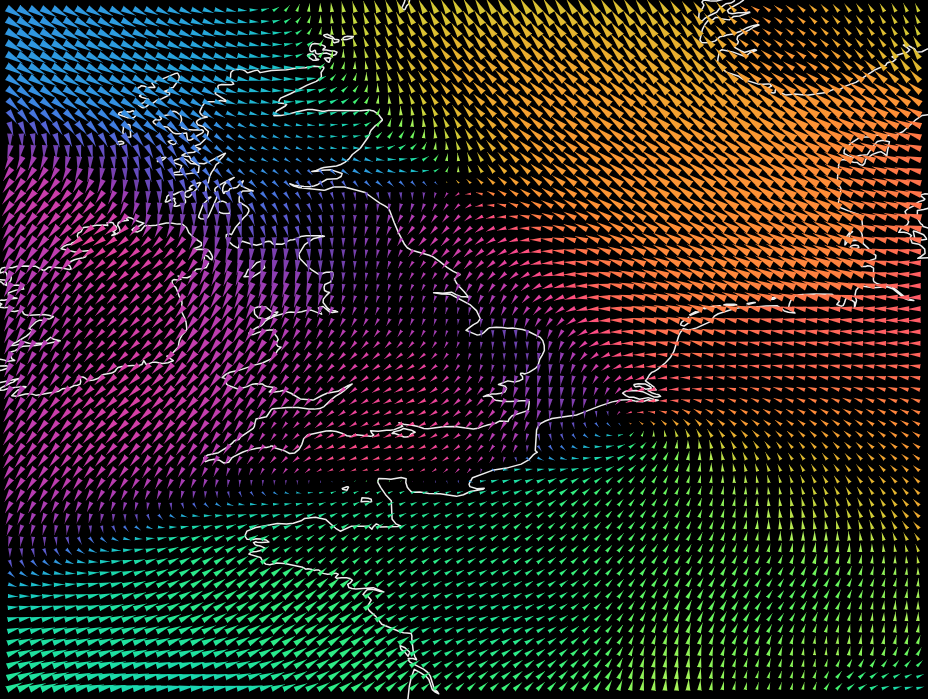
A vector field is a mathematical concept used in physics and engineering to describe a mapping of vectors (quantities with both magnitude and direction) to points in space. Each point in the space is associated with a vector, which typically represents some physical quantity such as velocity, force, or electromagnetic field strength. Vector fields are often visualized using arrows, with the direction of the arrow indicating the direction of the vector and the length of the arrow representing the magnitude of the vector at that point.
Purpose :
- Physics: In physics, vector fields are used to represent physical quantities that have both magnitude and direction, such as force, velocity, electric field, magnetic field, and gravitational field. Understanding the behavior of vector fields helps in analyzing and predicting the motion of particles, the interaction between charges and currents, and the propagation of waves.
- Engineering: Vector fields are crucial in engineering disciplines such as fluid dynamics, electromagnetism, structural mechanics, and aerodynamics. Engineers use vector fields to model and simulate complex systems, analyze the flow of fluids, design electromagnetic devices, optimize structures, and predict the behavior of mechanical systems.
- Mathematics: Vector fields are studied extensively in mathematics, particularly in the field of vector calculus and differential equations. Mathematicians analyze vector fields to understand their properties, study their behavior under different operations (such as divergence and curl), and solve differential equations governing physical phenomena.
- Computer Graphics: Vector fields are used in computer graphics and animation to simulate natural phenomena such as fluid flow, wind patterns, and particle motion. They play a crucial role in generating realistic visual effects, animating dynamic scenes, and creating immersive virtual environments.
- Robotics and Control Systems: Vector fields are applied in robotics and control systems for path planning, motion control, and robot navigation. Engineers use vector fields to guide the motion of robots, avoid obstacles, and achieve desired trajectories in both autonomous and teleoperated systems.
- Weather Forecasting: In meteorology and atmospheric science, vector fields are used to model and analyze weather phenomena such as wind patterns, air pressure gradients, and atmospheric circulation. Meteorologists use vector fields to simulate weather systems, track storm movements, and forecast local and global weather conditions.
- Geophysics and Geology: Vector fields are employed in geophysics and geology to study Earth’s magnetic field, seismic waves, and geological structures. Geophysicists use vector fields to map subsurface features, identify mineral deposits, and understand tectonic plate movements.
- Biology and Neuroscience: In biology and neuroscience, vector fields are used to represent biological processes such as fluid flow in blood vessels, electric field propagation in neurons, and cell migration in tissues. Understanding vector fields in biological systems helps in studying physiological functions, modeling biological phenomena, and developing medical treatments.
Uses :
- Fluid Dynamics: Vector fields are extensively used in fluid dynamics to represent fluid flow velocities. Understanding vector fields in fluid dynamics is essential for analyzing phenomena such as airflow around objects, ocean currents, turbulence, and heat transfer in fluids.
- Electromagnetism: In electromagnetism, vector fields are used to describe electric and magnetic fields. These fields play a crucial role in the behavior of charged particles, electromagnetic induction, and the operation of electrical devices such as motors, generators, and antennas.
- Mechanical Engineering: Vector fields are applied in mechanical engineering for stress analysis, deformation modeling, and structural design. Engineers use vector fields to visualize and analyze mechanical forces, displacement, and strain in solid structures under different loading conditions.
- Optics: In optics, vector fields are used to represent the polarization state of light. Polarization vector fields describe the orientation and amplitude of light waves, which is important in various optical applications such as polarimetry, optical imaging, and laser systems.
- Weather Forecasting: Meteorologists use vector fields to model atmospheric phenomena such as wind velocity, air pressure, and humidity. Vector fields are used in weather forecasting models to simulate weather patterns, predict storm tracks, and assess climate trends.
- Geophysics: Vector fields are employed in geophysics to study Earth’s magnetic field, gravitational field, and seismic waves. Geophysicists use vector fields to map subsurface structures, detect mineral deposits, and investigate the Earth’s interior composition and dynamics.
- Computer Graphics and Animation: Vector fields are used in computer graphics and animation to simulate natural phenomena such as fluid motion, smoke, fire, and hair dynamics. Artists and animators use vector fields to create realistic visual effects and dynamic simulations in movies, video games, and virtual reality environments.
- Robotics and Autonomous Vehicles: Vector fields are utilized in robotics for motion planning, obstacle avoidance, and navigation. Engineers use vector fields to guide the movement of robots, drones, and autonomous vehicles in complex environments, ensuring safe and efficient operation.
- Biophysics and Biomechanics: In biophysics and biomechanics, vector fields are used to study biological systems such as blood flow in vessels, muscle contractions, and cell movements. Understanding vector fields in biological systems helps in diagnosing diseases, designing medical devices, and predicting biological behavior.
- Mathematics and Physics Research: Vector fields play a fundamental role in mathematical and physical research, particularly in the study of differential equations, dynamical systems, and nonlinear phenomena. Mathematicians and physicists use vector fields to explore fundamental principles of nature and develop theoretical models for complex phenomena.
Only logged in customers who have purchased this product may leave a review.
Related products
-
- Sale!
Contours
-
$ 15Original price was: $ 15.$ 10Current price is: $ 10. - Add to cart
-
- Sale!
Parallel Sets
-
$ 15Original price was: $ 15.$ 10Current price is: $ 10. - Add to cart
-
- Sale!
Radial Cluster Tree
-
$ 15Original price was: $ 15.$ 10Current price is: $ 10. - Add to cart


Reviews
There are no reviews yet.